Lab Website Under Construction - Check For Updates
Hydrodynamic Quantum Analogs

A primary goal of our work is to understand the extent to which quantum phenomena may be recreated through classical pilot-wave hydrodynamics. Hydrodynamic Quantum Analogs (HQAs) is the nascent field of study initiated in 2005 by the discovery of Yves Couder and co-workers that a millimetric droplet may walk along the surface of a vibrating liquid bath through a resonant interaction with its own quasi-monochromatic wave field. Identifying the droplet as a self-propelling wave source differentiates these walking droplets, or `walkers', from the great majority of classical systems involving wave-particle interactions. By virtue of the coupling with their wave fields, walking droplets extend the range of classical mechanics to include certain features previously thought to be exclusive to the quantum realm, including quantized orbits, tunneling, wave-like statistics, and particle diffraction and interference patterns.
This system has attracted a great deal of attention from both the scientific community and the media, as it constitutes the first known and directly observable pilot-wave system of the form proposed by Louis de Broglie in 1926 as a rational, realist alternative to the Copenhagen Interpretation. Since the famous 1927 Solvay Conference, modern physics has, by and large, ascribed to the Copenhagen Interpretation, according to which the statistical theory of quantum mechanics (QM) is a complete description of physical reality, so that microscopic particles are intrinsically probabilistic. The quantum-like behavior of the walking droplets suggests a re-evaluation of de Broglie’s realist theory.
Here’s a couple of videos describing our research.
Through the Wormhole
(Documentary on the Science Channel)
Video by Dan Harris and John Bush summarizing some of our research
HIGHLIGHTED PROJECTS

Anderson localization of walking droplets
Abraham, A. J., Malkov, S., Ljubetic, F. A., Durey, M., Sáenz, P. J.
Submitted (2023) - arXiv
Understanding the ability of particles to maneuver through disordered environments is a central problem in innumerable settings, from active matter and biology to electronics. Macroscopic particles ultimately exhibit diffusive motion when their energy exceeds the characteristic potential barrier of the random landscape. In stark contrast, wave-particle duality causes electrons in disordered media to come to rest even when the potential is weak -- a remarkable phenomenon known as Anderson localization. Here, we present a hydrodynamic active system with wave-particle features, a millimetric droplet self-guided by its own wave field over a submerged random topography, whose dynamics exhibits localized statistics analogous to those of electronic systems. Consideration of an ensemble of particle trajectories reveals a suppression of diffusion when the guiding wave field extends over the disordered topography. We rationalize mechanistically the emergent statistics by virtue of the wave-mediated resonant coupling between the droplet and topography, which produces an attractive wave potential about the localization region. This hydrodynamic analog, which demonstrates how a classical particle may localize like a wave, suggests new directions for future research in various areas, including active matter, wave localization, many-body localization, and topological matter.

Emergent order in hydrodynamic spin lattices
Sáenz, P. J., Pucci, G. Turton, S.E., Goujon, A., Rosales, R.R., Dunkel, J., Bush, J.W.M.
Nature, 596, 58–62 (2021) (Movies)
Macroscale analogues of microscopic spin systems offer direct insights into fundamental physical principles, thereby advancing our understanding of synchronization phenomena4 and informing the design of novel classes of chiral metamaterials. Here we introduce hydrodynamic spin lattices (HSLs) of ‘walking’ droplets as a class of active spin systems with particle–wave coupling. HSLs reveal various non-equilibrium symmetry-breaking phenomena, including transitions from antiferromagnetic to ferromagnetic order that can be controlled by varying the lattice geometry and system rotation. Theoretical predictions based on a generalized Kuramoto model derived from first principles rationalize our experimental observations, establishing HSLs as a versatile platform for exploring active phase oscillator dynamics. The tunability of HSLs suggests exciting directions for future research, from active spin–wave dynamics to hydrodynamic analogue computation and droplet-based topological insulators.

A hydrodynamic analog of Friedel oscillations
Sáenz, P. J., Cristea-Platon, T., & Bush, J. W. M.
Science Advances, 6, 20, eaay9234, (2020) (PDF) (Movies)
We present a macroscopic analog of an open quantum system, achieved with a classical pilot-wave system. Friedel oscillations are the angstrom-scale statistical signature of an impurity on a metal surface, concentric circular modulations in the probability density function of the surrounding electron sea. We consider a millimetric drop, propelled by its own wave field along the surface of a vibrating liquid bath, interacting with a submerged circular well. An ensemble of drop trajectories displays a statistical signature in the vicinity of the well that is strikingly similar to Friedel oscillations. The droplet trajectories reveal the dynamical roots of the emergent statistics. Our study elucidates a new mechanism for emergent quantum-like statistics in pilot-wave hydrodynamics and so suggests new directions for the nascent field of hydrodynamic quantum analogs.
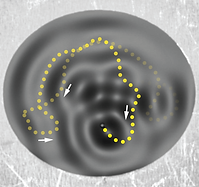
Statistical projection effects in a hydrodynamic pilot-wave system
Sáenz, P. J., Cristea-Platon, T., & Bush, J. W. M.
Nature Physics, 14, 315-319 (2018). (PDF)
Millimetric liquid droplets can walk across the surface of a vibrating fluid bath, self-propelled through a resonant interaction with their own guiding or ‘pilot’ wave fields. These walking droplets, or ‘walkers’, exhibit several features previously thought to be peculiar to the microscopic, quantum realm. In particular, walkers confined to circular corrals manifest a wave-like statistical behaviour reminiscent of that of electrons in quantum corrals. Here we demonstrate that localized topological inhomogeneities in an elliptical corral may lead to resonant projection effects in the walker’s statistics similar to those reported in quantum corrals. Specifically, we show that a submerged circular well may drive the walker to excite specific eigenmodes in the bath that result in drastic changes in the particle’s statistical behaviour. The well tends to attract the walker, leading to a local peak in the walker’s position histogram. By placing the well at one of the foci, a mode with maxima near the foci is preferentially excited, leading to a projection effect in the walker’s position histogram towards the empty focus, an effect strongly reminiscent of the quantum mirage. Finally, we demonstrate that the mean pilot-wave field has the same form as the histogram describing the walker’s statistics.
